basic leaf types
Whorled leaf pattern of the American tiger lily
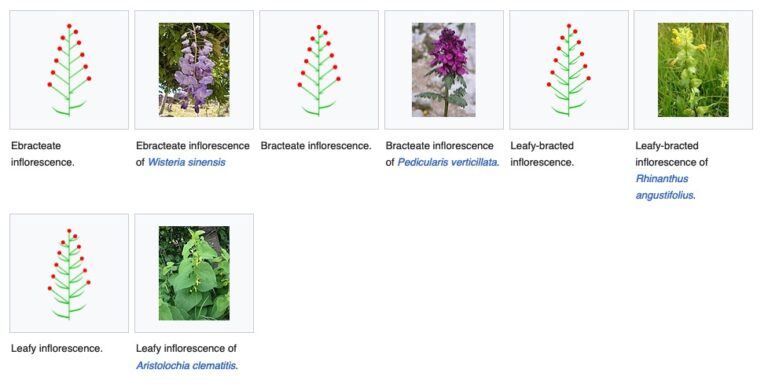
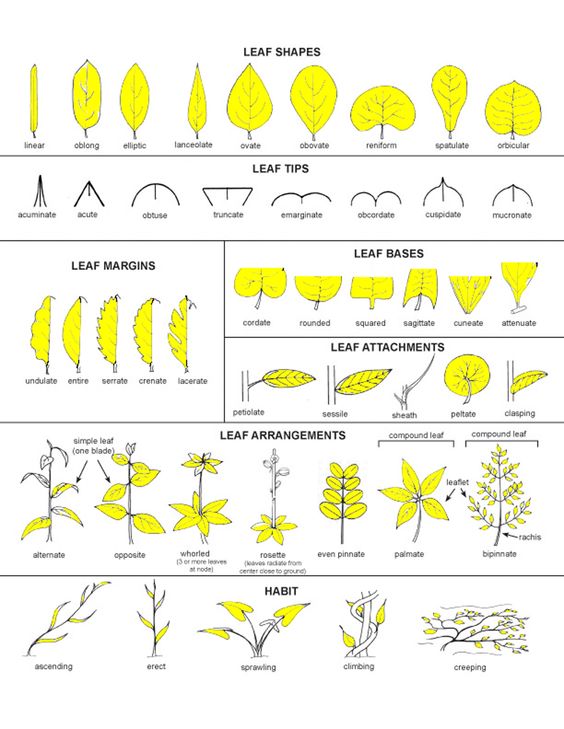
Perennial plants whose leaves are shed annually are said to have deciduous leaves, while leaves that remain through winter are evergreens. Leaves attached to stems by stalks (known as petioles) are called petiolate, and if attached directly to the stem with no petiole they are called sessile.[20]
- Ferns have fronds.
- Conifer leaves are typically needle- or awl-shaped or scale-like, they are usually evergreen, but can sometimes be deciduous. Usually, they have a single vein.
- Flowering plant (Angiosperm) leaves: the standard form includes stipules, a petiole, and a lamina.
- Lycophytes have microphylls.
- Sheath leaves are the type found in most grasses and many other monocots.
- Other specialized leaves include those of Nepenthes, a pitcher plant.
Dicot leaves have blades with pinnate vegetation (where major veins diverge from one large mid-vein and have smaller connecting networks between them). Less commonly, dicot leaf blades may have palmate venation (several large veins diverging from petiole to leaf edges). Finally, some exhibit parallel venation.[20]
Monocot leaves in temperate climates usually have narrow blades, and usually parallel venation converging at leaf tips or edges. Some also have pinnate venation.[20]
Arrangement on the stem
The arrangement of leaves on the stem is known as phyllotaxis.[21] A large variety of phyllotactic patterns occur in nature:

The leaves on this plant are arranged in pairs opposite one another, with successive pairs at right angles to each other (decussate) along the red stem. Note the developing buds in the axils of these leaves.

The leaves on this plant are alternately arranged (Senecio angulatus). Alternate One leaf, branch, or flower part attaches at each point or node on the stem, and leaves alternate direction, to a greater or lesser degree, along the stem. Basal Arising from the base of the stem. Cauline Arising from the aerial stem. Opposite Two leaves, branches, or flower parts attach at each point or node on the stem. Leaf attachments are paired at each node. Decussate An opposite arrangement in which each successive pair is rotated 90° from the previous. Whorled, or verticillate Three or more leaves, branches, or flower parts attach at each point or node on the stem. As with opposite leaves, successive whorls may or may not be decussate, rotated by half the angle between the leaves in the whorl (i.e., successive whorls of three rotated 60°, whorls of four rotated 45°, etc.). Opposite leaves may appear whorled near the tip of the stem. Pseudoverticillate describes an arrangement only appearing whorled, but not actually so. Rosulate Leaves form a rosette. Rows The term, distichous, literally means two rows. Leaves in this arrangement may be alternate or opposite in their attachment. The term, 2-ranked, is equivalent. The terms, tristichous and tetrastichous, are sometimes encountered. For example, the “leaves” (actually microphylls) of most species of Selaginella are tetrastichous, but not decussate.
In the simplest mathematical models of phyllotaxis, the apex of the stem is represented as a circle. Each new node is formed at the apex, and it is rotated by a constant angle from the previous node. This angle is called the divergence angle. The number of leaves that grow from a node depends on the plant species. When a single leaf grows from each node, and when the stem is held straight, the leaves form a helix.
The divergence angle is often represented as a fraction of a full rotation around the stem. A rotation fraction of 1/2 (a divergence angle of 180°) produces an alternate arrangement, such as in Gasteria or the fan-aloe Kumara plicatilis. Rotation fractions of 1/3 (divergence angles of 120°) occur in beech and hazel. Oak and apricot rotate by 2/5, sunflowers, poplar, and pear by 3/8, and in willow and almond the fraction is 5/13.[22] These arrangements are periodic. The denominator of the rotation fraction indicates the number of leaves in one period, while the numerator indicates the number of complete turns or gyres made in one period. For example:
- 180° (or 1⁄2): two leaves in one circle (alternate leaves)
- 120° (or 1⁄3): three leaves in one circle
- 144° (or 2⁄5): five leaves in two gyres
- 135° (or 3⁄8): eight leaves in three gyres.
Most divergence angles are related to the sequence of Fibonacci numbers Fn. This sequence begins 1, 1, 2, 3, 5, 8, 13; each term is the sum of the previous two. Rotation fractions are often quotients Fn / Fn + 2 of a Fibonacci number by the number two terms later in the sequence. This is the case for the fractions 1/2, 1/3, 2/5, 3/8, and 5/13. The ratio between successive Fibonacci numbers tends to the golden ratio φ = (1 + √5)//2. When a circle is divided into two arcs whose lengths are in the ratio 1:φ, the angle formed by the smaller arc is the golden angle, which is 1/φ2 × 360° ≈ 137.5°. Because of this, many divergence angles are approximately 137.5°.
In plants where a pair of opposite leaves grows from each node, the leaves form a double helix. If the nodes do not rotate (a rotation fraction of zero and a divergence angle of 0°), the two helices become a pair of parallel lines, creating a distichous arrangement as in maple or olive trees. More common in a decussate pattern, in which each node rotates by 1/4 (90°) as in the herb basil. The leaves of tricussate plants such as Nerium oleander form a triple helix.
The leaves of some plants do not form helices. In some plants, the divergence angle changes as the plant grows.[23] In orixate phyllotaxis, named after Orixa japonica, the divergence angle is not constant. Instead, it is periodic and follows the sequence 180°, 90°, 180°, 270°.[24]